Extreme superposition: rogue waves of infinite order and the Painlevé-III hierarchy
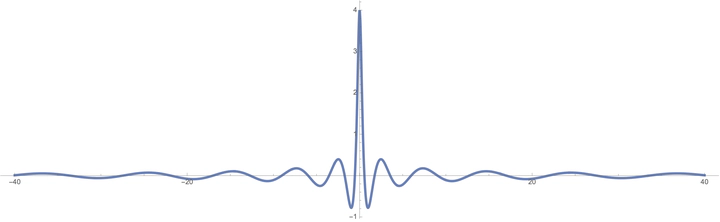The symmetric rogue wave of infinite order
Abstract
We study the fundamental rogue wave solutions of the focusing nonlinear Schrödinger equation in the limit of large order. Using a recently proposed Riemann–Hilbert representation of the rogue wave solution of arbitrary order $k$, we establish the existence of a limiting profile of the rogue wave in the large-$k$ limit when the solution is viewed in appropriate rescaled variables capturing the near-field region where the solution has the largest amplitude. The limiting profile is a new particular solution of the focusing nonlinear Schrödinger equation in the rescaled variables—the rogue wave of infinite order—which also satisfies ordinary differential equations with respect to space and time. The spatial differential equations are identified with certain members of the Painlevé-III hierarchy. We compute the far-field asymptotic behavior of the near-field limit solution and compare the asymptotic formulas with the exact solution using numerical methods for solving Riemann–Hilbert problems. In a certain transitional region for the asymptotics, the near-field limit function is described by a specific globally defined tritronquée solution of the Painlevé-II equation. These properties lead us to regard the rogue wave of infinite order as a new special function.
Type
Publication
Duke Mathematical Journal 169, 671–760, 2020
Below is an animation for the short-time evolution of rogue wave of infinite order $\Psi(X,T)$, a special solution of the focusing NLS equation
$$ \mathrm{i}\Psi_t + \frac{1}{2}\Psi_{XX}+|\Psi|^2\Psi=0, $$identified in this work. The computational method developed in this work is based on numerical solution of the Riemann-Hilbert problem representation of $\Psi(X,T)$ for each $(X,T)$. While this Mathematica module can be used in code repository linked above, we recommend using the more recent Julia software package RogueWaveInfiniteNLS.jl
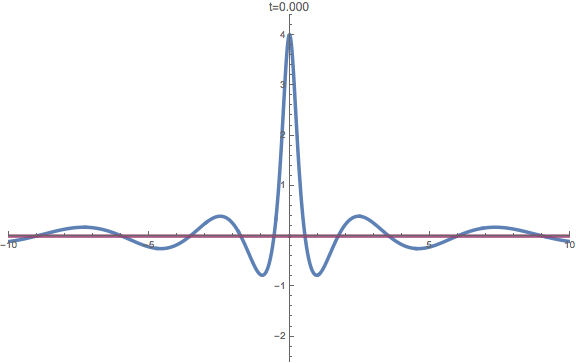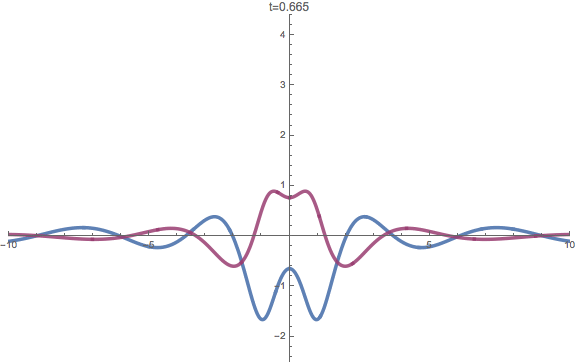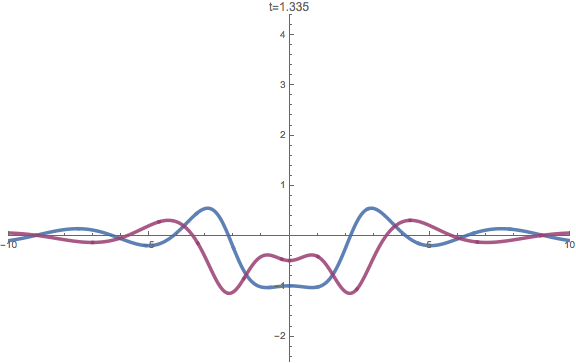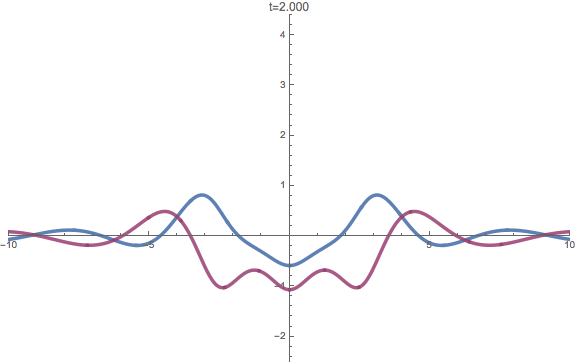
Time evolution of the special solution. Blue: the real part, purple: the imaginary part
