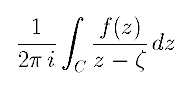Intro to Complex Analysis

(15-Math-6005)

Riemann sphere, Möbius transformations, Cauchy-Riemann equations, Cauchy's Theorems & Integral Formulas, Argument Principle, The Residue Theorem, Riemann Mapping Theorem, Schwarz Christoffel Formula

Department of

Mathematical

Sciences

This page is a work in progress! (Last revised 23 Nov 2020)

Instructor Prof David A Herron
4514 French Hall West 556-4075 		My Office Hours
TBA and online by appt 		E-mail me at David's e-address
My web page is at David's w-address

Basic Course Information

There are zillions of books about Complex Analysis available in the Geo-Math-Physics Library. Below I list some texts which I think are pretty good, although they mostly are more theoretical.

Text Book
For our class, I will follow A First Course in COMPLEX ANALYSIS with Applications by Zill and Shanahan; the third edition is available, although rather expensive and I suspect you can find (and use) earlier editions for much much less---please ask me if you cannot locate these.
Hint: look here.

General Syllabus
I hope to cover most of the text, plus possibly some additional material.

Sometimes it can be a good idea to look at more than one book/resource, because often some author will say things in just the 'right' way. Below are some books that I know or have heard good things about. The books by Ahlfors and Carathéodory are very geometric, which I quite like, but rather sophisticated. The book by Greene & Krantz is interesting and takes a novel approach. The books by McGehee and Palka are elementary with lotsa details presented. The books by Churchill & Brown, Greene & Krantz, McGehee, and Palka are all loaded with good problems. I had planned to use the book by Polya & Latta; it has some great parts too it, and you can get a pdf below.

Also, do not overlook the web as a resource. For example, see Links.

P Palka An Introduction to Complex Function Theory
CB Churchill & Brown Complex Variables and Applications
GK Greene & Krantz Function Theory of One Complex Variable (second edition)
M McGehee An Introduction to Complex Analysis
PL Polya & Latta Complex Variables
A Ahlfors Complex Analysis (third edition)
C Carathéodory Theory of Functions (of a complex variable) Vols. I & II (second edition)

Important Dates

Course Structure

While this is an online course, I recommend that you think of it as a traditional MWF class. Each week we will cover the sections in the text as described in the Weekly Syllabus. For each section, you should review the text material, watch the associated videos, and work the suggested exercises; again, see the Weekly Syllabus. Also, there will be assigned/collected/graded homework that covers the recent material. Please see....

An online course requires a different approach from a traditional in-class course. While you spend no time commuting to UC and no time in a classroom, the lack of one-on-one interaction with the instructor requires extra effort studying the course videos and textbook. In order to fully understand the concepts being presented, you must actively watch the videos, and you may need/want to repeatedly reread the text and/or rewatch the videos.

Each week I will post a number of short videos (on EdPuzzle, the online platform we'll be using for video lectures---see the Course Tools) that will discuss the material. Here are a few important facts about the videos on EdPuzzle: I also plan a weekly online problem session. Initially this will be me answering your questions and possibly working through some examples. However, once we work out some details, each of you will be asked to present solutions to problems. During the first week of class we will determine a time that we can all meet (online).

Course Goals

The main course objective is to learn about complex numbers and especially complex variable calculus. A second objective is to understand some geometry in Euclidean $2$-dimensional space $\mathbb{R}^2$ and some physical applications. Here is a brief list of some of the topics we will cover: 3 ways to view and use complex numbers, complex arithmetic, complex valued functions, elementary mappings, complex differentiablity, the Cauchy-Riemann equations, complex path integrals, Cauchy's theorem and integral formulas, Taylor and Laurent series, the residue theorem, conformal mappings

Course Tools

Here are the major platforms that you need to become familiar with:

Your Course Grade

Your final grade will be based on homework assignments, video watching/answering questions, and problem session participation. Here is a potential breakdown (which may be revised): Your grade will be determined solely from the above data---there will not be any possible "extra credit".

Homework will be assigned daily (see the Weekly Syllabus), and there will also be HW sets that are collected and graded. I encourage you to work with other members of the class, but the HW that you turn in must be your own work. It is your responsibility to turn in the homework assignments on or before the due dates. Late homework will not be accepted. Please adhere to the guidelines given below (at the very end) when writing your assignments. Work which does not meet these requirements will not be graded.

Unless I explicitly indicate otherwise, you should read everything in the text and try to work all of the exercises and problems that you find as you read. Any of these exercises, as well as all of the "fill in the details" that I mention during lectures, are fair game as "easy" exam questions.

Each week (or so) I will pass out a set of problems, many chosen from the text book. I will ask you write up and hand in solutions to certain of these exercises (aka, HomeWork); these will be graded and returned to you. Please be sure to check out my guidelines for writing up your HW solutions.

In order to receive audit credit for this course, you must attend all lectures and take all quizzes and exams.

I encourage you to talk to other members of the class or to ask me for help.

Course Exams

Due to the unusual and crazy circumstances surrounding the upcoming semester, there will be no in class exams. However, sometime during Final Exams week, each of you will take an oral exam. This will most likely be online, and I will say more about it later.

Weekly Syllabus

 Here is a detailed syllabus along with some suggested homework. This is based on the first edition of the text. I encourage you to work the suggested exercises from each section, but this will not be graded.

Week ofMaterial Covered Suggested ExercisesRemarks
Aug 24Sections 1.1, 1.2, 1.3, 1.4 Section 1.1: 9, 10, 14, 25, 27, 30 31, 33, 41, 45, 51
Section 1.2: 5, 7, 11, 13, 15, 17, 21, 25, 31, 34, 37, 41
Section 1.3: 7, 9, 13, 19, 23, 27, 35, 37, 41, 43, 45, 47
Section 1.4: 7, 14, 17, 27, 31, 33
Aug 31Sections 1.5, 2.1, 2.2, 2.3, 2.4 Section 1.5: 3, 7, 11, 17, 25, 29, 35, 37, 45, 47-49
Section 2.1: 11, 13, 15, 27(a,c), 29(b), 33
Section 2.2: 5, 8, 13, 14, 17, 24, 27, 31, 33
Section 2.3: 5, 11, 12, 15, 21, 23, 25, 31, 35, 37
Section 2.4: 11, 13, 17, 21, 23, 29, 37, 41, 47, 49, 51
Week of Material CoveredSuggested Exercises Remarks
Sep 7Sections 2.5, 2.6 Section 2.5: 3, 9, 13, 15, 17, 19, 21, 27
Section 2.6: 11, 13, 17, 33, 37
Chapter 1 Review Quiz: 1-45 (odd)
Chapter 2 Review Quiz: 1-33 (odd)		 No class Monday
Labor Day
Sep 14 Sections 3.1, 3.2, 3.3 Section 3.1: 5, 15, 19, 21, 35
Section 3.2: 5, 11, 15, 17, 21, 25
Section 3.3: 4, 7, 13, 17, 18
Sep 21 Sections 3.4, 4.1 Section 3.4: 2, 3, 4, 7, 9, 13
Chapter 3 Review Quiz: 1, 6, 7, 8, 10, 11, 12, 13, 19
Section 4.1: 5, 7, 11, 15, 17, 19, 23, 31, 35, 43, 45, 51, 52, 55
Sep 28 Sections 4.2, 4.3, 4.4 Section 4.2: 3, 5, 11, 17, 21, 22, 24
Section 4.3: 5, 9, 11, 12, 25, 28, 31, 39, 43
Week of Material CoveredSuggested Exercises Remarks
Oct 5 Sections 4.4, 5.1, 5.2 Section 4.4: 3, 5, 11, 13, 21
Chapter 4 Review Quiz: 6, 7, 8, 12, 28, 32
Section 5.1: 9, 11, 17, 19, 25, 27, 33
Section 5.2: 3, 5, 7, 9, 15, 19, 20, 23, 25, 27, 33		  
Oct 12 Sections 5.3, 5.4 Section 5.3: 5, 9, 11, 17, 21, 25, 27, 29, 31
Section 5.4: 12, 15, 20		 No class Monday
Reading Day
Oct 19 Sections 5.4, 5.5, 5.6 Section 5.4: 12, 15, 19, 20, 25, 27, 28
Section 5.5: 5, 9, 11, 17, 19, 21, 23, 25, 27(c), 33
Section 5.6: 8, 9, 25, 26
Chpt 5 Review Quiz: 1-19 (odd), 25, 27, 29, 34, 37		  
Oct 26Sections 6.1, 6.2 Section 6.1: 13, 19, 23, 25, 27, 29, 39, 41, 43, 45
Section 6.2: 1, 4, 13, 15, 19, 25, 27, 31, 49
Week of Material CoveredSuggested Exercises Remarks
Nov 2 Sections 6.3, 6.4 Section 6.3: 3-21 (odd), 27, 31, 33
Nov 9 Sections 6.4, 6.5 Section 6.4: 2, 8, 9, 10, 12, 13, 21, 22, 24, 27, 29, 30, 31, 33, 34
Section 6.5: 5, 13, 16, 19, 25, 33, 37, 42		 No class Wednesday
Veteran's Day
Nov 16 Sections 6.6 Section 6.6: 3, 7, 13, 15, 23, 29, 40, 43, 46, 51, 59, 61, 65
Nov 23 Sections 7.1, 7.2 Section 7.1: 1, 3, 13, 14, 17, 19 No class Friday
Black Friday!
Nov 30 Sections 7.2, 7.3 Section 7.2: 3, 7, 11, 14, 15, 23, 25, 28, 29
Section 7.3: TBA		 Last week!
Week of Material CoveredSuggested Exercises Remarks
Dec  7 Finals Week Final Exam Monday Dec 7 Final Exams week

Links

Here are some links to various pdf files for old notes, problems, text books, interesting web pages, etc.

Here are some links to articles about writing mathematics.

Writing Mathematics

Class Stuff

University Information

The last day to drop this class and last day to withdraw from this class are official UC dates and something I have no control over. If you withdraw from this course, I will be required to verify whether or not you minimally participated in the class. Although I will try my best to respond accurately, in the absence of any evidence to the contrary, I will state that you did not minimally participate. Ways for you to provide clear evidence of your presence in the class include turning in at least one homework assignment, taking at least one quiz, or taking at least one exam.

Academic Integrity Policy
The University Rules, including the Student Code of Conduct, and other documented policies of the department, college, and university related to academic integrity will be enforced. Any violation of these regulations, including acts of plagiarism or cheating, will be dealt with on an individual basis according to the severity of the misconduct.

Special Needs Policy
If you have any special needs related to your participation in this course, including identified visual impairment, hearing impairment, physical impairment, communication disorder, and/or specific learning disability that may influence your performance in this course, you should meet with the instructor to arrange for reasonable provisions to ensure an equitable opportunity to meet all the requirements of this course. At the discretion of the instructor, some accommodations may require prior approval by Disability Services.

Except for a few courses, all mathematics classes satisfy the University Quantitative Reasoning Requirements. This course satisfies the QRR of UC's General Education program. This course was designed following the guidelines of the University of Cincinnati General Education Program. It satisfies, or partially satisfies, the Quantitative Reasoning distribution requirement. Moreover, of the five Baccalaureate Competencies, this course focuses on Critical Thinking, Effective Communication, and Information Literacy.