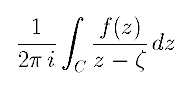Complex Analysis (15-Math-601)	Riemann sphere, Möbius transformations, Cauchy-Riemann equations, Cauchy's Theorems & Integral Formulas, Argument Principle, The Residue Theorem, Riemann Mapping Theorem, Schwarz Christoffel Formula	Department of Mathematical Sciences

This page is a work in progress! (Last revised 25 September 2009)

Textbooks There are zillions of books about Complex Analysis available in the Geo-Math-Physics Library. I have put a number of these on open reserve---if you check one out at the end of the day, you can keep it over night. Below is a short list of texts which I think are pretty good. For the most part, I will follow Ahlfors. The books by Carathéodory are very geometric, which I quite like. The book by Greene & Krantz is interesting and takes a novel approach. The books by McGehee and Palka are elementary with lotsa details presented. The books by Greene & Krantz, McGehee, and Palka are all loaded with good problems.

A	Ahlfors	Complex Analysis (third edition)
C	Carathéodory	Theory of Functions (of a complex variable) Vols. I & II (second edition)
GK	Greene & Krantz	Function Theory of One Complex Variable (second edition)
M	McGehee	An Introduction to Complex Analysis
P	Palka	An Introduction to Complex Function Theory

General Syllabus Chapters: 1-4 in A; 1-3,6 in GK; 1-3 in M; I-V,IX in P

Course Goals First and foremost, this is a course geared towards teaching you to think, act, and problem solve like a mathematician. Of particular importance, to me, is your acquiring the ability to communicate mathematical ideas effectively. This means learning how to read, and especially to write, mathematical proofs. We shall accomplish this in the guise of studying elementary complex analysis and the roots of geometric function theory. A subgoal, at least for some of you, is preparation for the preliminary PhD examination in complex analysis; I will have more to say about this in spring quarter (at which time I will run a prelim practice problem session).

Grades Your final course grade will be determined from your performance on midterm exams, a final exam, your homework scores, and class and problem session participation. Roughly speaking, an A means your work is at the PhD level, a B indicates masters level work, and anything less describes work which is not at the graduate level.

Homework & Problem Session I have a long list of suggested problems for you to solve. I plan to have a weekly hour long (90 minutes if possible:-) problem session. Here I will expect you, the students, to present solutions to certain of the homework problems. We can also talk about other problems and/or topics from the lectures. On the first day of class we will decide when to hold the problem session. I will also ask you write up and hand in solutions to certain exercises; these will be graded and returned to you. See below for due dates. I'll say more about this in class.

Problem Session Mon 1:00-1:50 room TBA

Below I list information regarding: this week's hot topics, some suggested problems from Ahlfors, and homework.

Here are suggested problems for each indicated section in Ahlfors. In general you should try to solve all of the problems in Ahlfors, especially if you are planning to take the preliminary examination in Complex Analysis; but, be aware that some of Ahlfors' problems require some ingenuity. As I mentioned above, the other books also have many many good problems, many of which are routine.

• I.1.1, Complex Arithmetic, pp.2-3:1,2
• I.1.2, Square Roots, p.4:3,4
• I.1.4, Conjugation & Absolute Value, p.9:3,4
• I.1.5, Inequalities, p.11:1,3,4
• I.2.1, Geometric Arithmetic, p.15:1-3
• I.2.2, Roots, p.16:3
• I.2.3, Analytic Geometry, p.17:1-3,5
• I.2.4, Riemann Sphere, p.20:1,2,4,5
• II.3.1-2,Complex Exp & Trig Functions, p.44:1,2,4
• II.3.3-4, Complex Logarithm & Powers, p.47:3-8
• III.1.1-2, Metric Topology, p.53:(1,2),3,4,6,7
• III.1.3, Connectedness, p.58:2,3,6
• III.1.4, Compactness, p.63:2,3,4
• III.1.5, Continuity, p.66:1-5
• II.1.2, Holomorphic Functions, p.28:1-7
• III.2.2, Holomorphic Functions in Regions, p.72:1-3
• III.2.3, Conformal Mapping, read pp.73-75
• III.3.1, The Möbius Group, p.78:1-4
• III.3.2, The Cross Ratio, p.80:1-4
• III.3.3, Symmetry, pp.82-83:1-8
• III.4.2, Elementary Mappings, pp.96-97:1-8

Below is the assigned homework with due dates. (Here, again is a link to a pdf file for a list of these homework problems). The "Problems To Hand In" are to be written up and handed in before class on the indicated dates. The "For Session" problems will be discussed in that week's problem session. After the due date, you can find outlines for solutions (at least for some of the problems) by clicking the appropriate problem number.

Due Dates	Problems To Hand In			For Session
23,25 Sept	No Class	First Day	4	No Session
28,30 Sept; 2 Oct	7	9	12(a)	2--10
5,7,9 Oct	23	24	22	14-21, 25-29
12,14,16 Oct	34	36	32,39	29-31,33,35,37,38,41,42
19,21,23 Oct	44	54	46	37,43,47,48,53,55
26,28,30 Oct	67	60	72,73	58,61,62,64-66,68-72
2,4,6 Nov	MidTerm		75	No Session
9,11,13 Nov	78	Vets Day	87,88	77,80,83,86, Ahlfors/p.72/2,3
16,18,20 Nov	90	93	95,98	83,91,92,94,96,97
23,25,27 Nov	100,103,106 all due Wed		Trky Day	94,99,101,102,104,105
30; 2,4 Dec	104, 109, 124 all due Fri			(16,17,27),30,31,94,101,108,111-113
11 Dec	Final Exam 1:30-3:30			114-129