The 2011 Ohio River Analysis Meeting

We show the L^p boundedness of wave operators in R³ for a scaling-invariant class of potentials. This is a consequence of a structure formula, which can also be used to prove several other results concerning wave operators and, by extension, the perturbed Schrödinger or wave evolution.

Oleksandra Beznosova (Missouri): The limiting case of the Reverse Hölder inequalities and the A_∞ condition.

As  p → 1,  p-Reverse Holder inequalities for a weight degenerate to a reverse Jensen's inequality of the L log L type. We show that the constant in this inequality depends at most linearly on the A_∞ constant of the weight .

This is a joint work with Sasha Reznikov.

We consider infinite-volume quantum harmonic lattice systems and study the decay of certain time-evolved observables in the large-time regime. The decay rate depends on the support of an observable and the on-site space dimension.

The small-dispersion or semiclassical sine-Gordon equation models magnetic flux propagation in long Josephson junctions. For a broad class of pure impulse initial data, the leading-order solution is given by modulated elliptic functions describing superluminal kink trains and breather trains at small times. This justifies the formal results obtained from Whitham averaging. We will describe the solution at transition points between the kink and breather trains in terms of Painleve functions. This is joint work with Peter Miller.

We prove that the bound for a general Haar shift operator is linear with respect to the A₂ "norm" of the weight. It has been shown in many different ways, for instance, corona decomposition and local mean oscillations. Here we establish the linear weighted norm estimate using dyadic approximation and an iterated Bellman function method.

We will prove that solutions to the supercritical dissipative surface quasi-geotrophic equation eventually become smooth by examining the evolution of the equation on a test class of functions that is dual to Holder C^β functions. Using induction on scales, we will prove that there is a finite time after which the solution gains a certain degree of Holder continuity, which turns out to be a sufficient condition for smoothness.

I'll describe a new type of estimates for paraproducts and its application to time-frequency analysis. Joint work with Camil Muscalu and Christoph Thiele.

We study W^1,p estimates in Lipschitz domains for second order elliptic equations of divergence form with real-valued, bounded, measurable coefficients. For any fixed p>2, we prove that a weak reverse Hölder inequality implies the W^1,p estimates for solutions with Neumann boundary conditions. As a result, we are able to show that if the coefficient matrix is symmetric and uniformly continuous, the W^1,p estimate holds for   3/2-ε  <  p <  3+ε   if n ≥ 3, and for 4/3-ε  <  p <  4+ε   if n = 2.

We study the integral equation u=B_α(u^p) on Rⁿ, in which B_α is the Bessel potential. The regularity estimates of the positive solutions for the equation are proved. Precisely, given proper integrability assumption of the solutions, we show that they are uniformly bounded and Lipschitz continuous.

We establish both local and global well-posedness of the heat flow of polyharmonic maps from Rⁿ to a compact Riemannian manifold without boundary for initial data with small BMO norms.

We will describe some joint work with V. G. Maz'ya and I. E. Verbitsky concerning homogeneous linear and quasilinear differential operators. The model operator under consideration is

L(u) = -div(A)u - σ u

Here A is a uniformly elliptic, bounded measurable matrix function; and σ is a signed measure, or more generally a distribution. An approach to studying the operator L under only necessary conditions on σ will be discussed. These results have application to the characterization of certain L² Sobolev inequalities with indefinite weight.

In addition we will briefly discuss a quasilinear analogue of the operator L above, from which new characterizations of L^p Sobolev inequalities with indefinite weight can be deduced.

Localized energy estimates for the wave equation on Minkowski and (1+3)-dimensional Schwarzschild space-times have had various applications; for example, in the proof of Price's Law. We discuss a similar localized energy estimate for the homogeneous wave equation □φ = 0 on the (1+n)-dimnsional hyperspherical Schwarzschild manifold.

In this talk I will discuss joint work with Kaj Nyström on regularity and free boundary regularity type problems for positive p-harmonic functions,  p fixed, 1 < p < ∞, vanishing on a portion of a Reifenberg flat or NTA Ahlfors regular domain. This paper can be considered as a generalization to more general domains of our work on C¹ and Lipschitz domains, as well as the p=2 harmonic case of work of Kenig and Toro.

Rigidity of functions whose gradient lies in a subject of the conformal matrices is a classic subject, the one of the earliest theorems being Liouville's Theorem that conformal mappings in R³ are either affine or Mobius. There has been extensive work on developing a quantitative generalization of this result, one of the best known theorems has been Reshetnyak's compactness theorem that says if u_n is a weakly converging sequence in W^1,1 and   ∫ dist(Du,SO(n)) dx → 0 then u_n converges strongly in W^1,1 to an affine map whose gradient is a rotation. We reformulate this theorem as a special case of the question: What happens when two sequences of functions have increasingly similar symmetric part of gradient? We will provide a sharp answer to this question and consequently present a broad generalization of Reshetnak's theorem.

We will discuss sufficient conditions on pairs of weights  (u, v)  for commutators of classical operators to be bounded from L^p(v) to L^p(u). Our results are sharp and they demonstrate that commutators are more singular than the underlying operator. This work is based on a collaboration with David Cruz-Uribe.

Volberg and Treil recently showed that the norm of every classical Calderón-Zygmund Operator in a weighted space L²(w) is controlled by the first power of the A₂-norm of the weight. I'll present a simplified proof of the main step in their argument.

In this talk I will discuss the mixed problem or Zaremba's problem for the Laplacian in bounded Lipschitz domains of dimension two or higher. The boundary of the domain is decomposed into Neumann and Dirichlet sets which are disjoint. We specify conditions on the domain, N and D, as well as on the boundary data, so that the mixed problem has a unique solution whose non-tangential maximal function of the gradient belongs to the space L^p of the boundary. This is joint work with Justin Taylor and Russell Brown.

Last year saw the solution of the A₂-conjecture by Tuomas Hytönen: every Calderón-Zygmund operator obeys a linear bound on L²(w) with respect to the A₂-characteristic of the weight. Over a span of 8 years starting in 2000, one a time this was proved for a few operators that where either dyadic to begin with, or could be realized as suitable averages of what we now call Haar shift operators of bounded depth (very specific ones), and each one of these linear bounds was proved using Bellman function techniques. The last two years different techniques were brought to the table that will now handle the whole family of Haar shift operators, and more importantly averaging techniques which allowed finally to cover the whole class of Calderón-Zygmund singular integral operators. Notable are the contributions from Lacey-Petermichl-Reguera, CruzUribe-Martell-Pérez, and Pérez-Treil-Volberg, and Hytönen brilliantly put together all these ingredients plus some magic touch and was able to finish the argument.

The commutator of a Calderón-Zygmund singular integral operator with a BMO function is known to be more singular than the operator itself. This can be quantified by its lack of weak-type (1,1) estimates, and by the fact that the commutator is dominated by  M² instead of  M (where  M is the Hardy-Littlewood maximal operator). It can be also quantified in terms of the growth of the operator bound in weighted Lebesgue spaces. In joint work with Carlos Pérez and Daewon Chung we showed that if a linear operator obeys a linear bound in L²(w) with respect to the A₂-characteristic of the weight, then the commutator obeys a quadratic bound. In light of Hytönen's result, this behavior is shared by all Calderón-Zygmund singular integral operators. Our proof is based in the classical Coifman-Rochberg-Weiss argument. However, the first results in this direction (somehow mimicking the history of the A₂-conjecture), were due to Daewon Chung. In his PhD dissertation Chung proved, using Bellman function techniques, that the quadratic bound will hold and be sharp for the commutator of the Hilbert transform, and for Haar shift operators, and therefore for the Riesz and Beurling transforms. In this talk we will present these results, give some background and give a roadmap for the proofs.

The Davey-Stewartson II (DS II) equation is a completely integrable, nonlinear dispersive equation in two space and one time dimensions which models weakly nonlinear surface waves in a fluid of shallow depth. We study the scattering map for the linear system associated to the DS II equation and use it to show that the defocussing Davey-Stewartson II equation is globally well-posed in the space H^1,1(R²) consisting of L²(R²) functions  f(z)  with z and  |z| f(z)  also in L². We will explain why this result appears to be optimal and discuss prospects for studying the dynamics of the DS II equation, including temporal asymptotics and stability of solitons, in depth.

We study a class of initial boundary value problems of the KdV equation posed on a finite interval with nonhomogeneous boundary conditions. The IBVP is known to be locally well-posed, but its global L²-a priori estimate is not available and therefore it is not clear whether its solutions exist globally or blow up in finite time. We proved that the solutions exist globally as long as their initial value and the associated boundary data are small, and moreover, those solutions decay exponentially if their boundary data decay exponentially.

In some physical problems, such as granular media, sedimentation, and magma dynamics, the leading order equations are degenerately dispersive. A rigorous analysis of such equations has only recently begun and remains incomplete. Though some cases are locally, and globally, well-posed, others may be ill-posed.

In this talk, we consider the Rosenau-Hyman compacton equations. Inspired by a proof of ill-posedness for a surrogate equation, we present robust numerical evidence that the K(2,2) compacton equation is ill-posed. This is done by constructing a family of initial conditions for a regularized equation. As both the regularization parameter and the initial conditions vanish, the solution, at a fixed T > 0, becomes "large." Thus, the equations may be ill-posed in the sense that the solution operator ceases to be continuous about the zero solution.

Let u be a solution to the equation □_gu = 0 where g is some (nonstationary) Lorentzian metric and □_g its associated d'Alembertian. If we assume a priori that certain local energy norms for u and its higher derivatives hold, we can prove that u decays pointwise like t⁻³⁺. As an application, we can prove the aforementioned decay on Kerr spacetimes and some perturbations. This is joint work with Jason Metcalfe and Daniel Tataru.

Some Hessian inequalities for k-convex functions will be discussed, including fully nonlinear analogues of the Sobolev and Poincaré inequalities, and relations berween the k-Hessian energy   E_k[u] = ∫_Ω -uF_k [u dx],  Ω 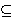Rⁿ, and the fractional Laplacian energy associated with (-Δ)^k/(k+1). Here F_k (k = 1,...,n) is the k-Hessian operator, i.e. the sum of all the k × k principal minors of the Hessian matrix of  u.

We present results on bound states and scattering properties for linear and nonlinear Schrödinger equations with oscillatory coefficients and explain the connection to some problems in fundamental and applied physics concerning the propagation of waves through microstructures.