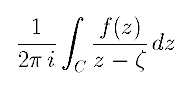Complex Analysis III

(15-Math-603)

Riemann sphere, Möbius transformations, Cauchy-Riemann equations, Cauchy's Theorems & Integral Formulas, Argument Principle, The Residue Theorem, Riemann Mapping Theorem, Schwarz Christoffel Formula

Department of

Mathematical

Sciences

This page is a work in progress! (Last revised 5 April 2010)

Instructor Prof David A Herron
810D Old Chem Bldg, 556-4075 		My Office Hours
Mon, Wed 1:00-2:00 and by appt 		Link to a list of problems. Prelim Practice Session
Thursday 11:00-12:30 804 Old Chem

Textbooks There are zillions of books about Complex Analysis available in the Geo-Math-Physics Library. I have put a number of these on open reserve---if you check one out at the end of the day, you can keep it over night. Below is a short list of texts which I think are pretty good. For the most part, I will follow Ahlfors. The books by Carathéodory are very geometric, which I quite like. The book by Greene & Krantz is interesting and takes a novel approach. The books by McGehee and Palka are elementary with lotsa details presented. The books by Greene & Krantz, McGehee, and Palka are all loaded with good problems.
A Ahlfors Complex Analysis (third edition)
C Carathéodory Theory of Functions (of a complex variable) Vols. I & II (second edition)
GK Greene & Krantz Function Theory of One Complex Variable (second edition)
M McGehee An Introduction to Complex Analysis
P Palka An Introduction to Complex Function Theory

General Syllabus in A: chpt 4, 1-5, chpt 5, 1,5; chpt 6, 1,2; in GK: chpts 2-6, 8; in P: chpts IV-V, VII-IX

Course Goals First and foremost, this is a course geared towards teaching you to think, act, and problem solve like a mathematician. Of particular importance, to me, is your acquiring the ability to communicate mathematical ideas effectively. This means learning how to read, and especially to write, mathematical proofs. We shall accomplish this in the guise of studying elementary complex analysis and the roots of geometric function theory. A subgoal, at least for some of you, is preparation for the preliminary PhD examination in complex analysis; I will have more to say about this in spring quarter (at which time I will run a prelim practice problem session).

Grades Your final course grade will be determined from your performance on midterm exams, a final exam, your homework scores, and class and problem session participation. Roughly speaking, an A means your work is at the PhD level, a B indicates masters level work, and anything less describes work which is not at the graduate level.

Homework & Problem Session I have a long list of suggested problems for you to solve. I plan to have a weekly hour long (90 minutes if possible:-) problem session. Here I will expect you, the students, to present solutions to certain of the homework problems. We can also talk about other problems and/or topics from the lectures. On the first day of class we will decide when to hold the problem session. I will also ask you write up and hand in solutions to certain exercises; these will be graded and returned to you. See below for due dates. I'll say more about this in class.

Prelim Practice Session Thursday 11:00-12:30 804 Old Chem

Below I list information regarding: this week's hot topics, some suggested problems from Ahlfors, and homework.

29 March-2 April This week we begin our final examination of Cauchy Theory. We'll look at some algebra (homology theory) that simplifies certain topological considerations in our proof of Cauchy's Theorem. We also learn about simply connected and multiply connected domains, and homology bases.

5-9 April We start this week by proving Cauchy's Theorem and Integral Formula. Then we begin our discussion of Residue Theory.

12-16 April This week we complete the proof of the Residue Theorem, explain how to calculate residues for poles, reprove the Argument Principle, and learn how to calculate certain (often improper) real integrals by using residues.

19-23 April This week we learn about normal families and equicontinuity. We prove a version of the Arzela-Ascoli Theorem, and then Montel's Theorem. We also begin a discussion of the Riemann Mapping Theorem focusing on the key ideas involved in its proof.
26-30 April This week we prove the Riemann Mapping Theorem.
3-7 May This week we learn about the Schwarz Reflection Principle and do some applications. Then we start on the Schwarz Christoffel Formula.
10-14 May This week we derive the Schwarz Christoffel Formula.
17-21 May This week we .
24-28 MayThis week we .
2,4 June During our last week we .

Here are suggested problems for each indicated section in Ahlfors. In general you should try to solve all of the problems in Ahlfors, especially if you are planning to take the preliminary examination in Complex Analysis; but, be aware that some of Ahlfors' problems require some ingenuity. As I mentioned above, the other books also have many many good problems, many of which are routine.

Below is the assigned homework with due dates. (Here, again is a link to a pdf file for a list of these homework problems). The "Problems To Hand In" are to be written up and handed in before class on the following Monday. The "Prelim Practice Session" problems (indicated by year and J for June or S for Sept) will be discussed in that week's session. After the due date, you can find outlines for solutions (at least for some of the problems) by clicking the appropriate problem number.
Due Dates Problems To Hand In Prelim Practice Session
29,31,2 Mar,Apr First Day A/p.133/#4, A/p.154/#2, 7, 10 J/90 1,3,4,5 ; S/90 2,5
5,7,9 April 13, 16, 23, 24 S/90 5; J/91 1,2,3 ; S/91 1,2,5
12,14,16 April Due on 21 April -- 28 S/91 3,5; J/92 2,3,4,5
19,21,23 April J/90 2; S/90 3; S/91 4,5; J/92 1,4; S/92 1-5
26,28,30 April Due on 30 April -- 31,33,36, Ahlfors/p.227/#3 Sept/92 ; S/91 - 1 ; J/91 - 5
3,5,7 May J/91 - 5; S/91 - 5; S/92 - 4; J/93 - 1-5
10,12,14 May Due on 17 May -- 42,44,47,51, Ahlfors/p.97/#5,#8 J/91 - 5; S/91 - 5; S/92 - 4; J/93 - 4,5; S/93 all
17,19,21 May
24,26,28 May
2,4 June Mem'l Day Final Review
7 June Final Exam 9:45-11:45